Let Us Understand What Is Binomial Coefficient.
the binomial coefficient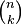 is the coefficient of the x k term in the polynomial expansion of the binomial power (1 + x) n.
is the coefficient of the x k term in the polynomial expansion of the binomial power (1 + x) n.
A binomial coefficient equals the number of combination of r items that can be selected from a set of n items. It also represents an entry in Pascal's triangle. These numbers are called binomial coefficients because they are coefficients in the binomial theorem.
Example:

the binomial coefficient
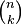 is the coefficient of the x k term in the polynomial expansion of the binomial power (1 + x) n.
is the coefficient of the x k term in the polynomial expansion of the binomial power (1 + x) n.A binomial coefficient equals the number of combination of r items that can be selected from a set of n items. It also represents an entry in Pascal's triangle. These numbers are called binomial coefficients because they are coefficients in the binomial theorem.
Example:






 notation avoids confusion with the notation for the conjugate transpose of a matrix, which can be thought of as a generalization of complex conjugation. If a complex number is represented as a 2×2 matrix, the notations are identical.We also can use scientific notation converter to find the number.
notation avoids confusion with the notation for the conjugate transpose of a matrix, which can be thought of as a generalization of complex conjugation. If a complex number is represented as a 2×2 matrix, the notations are identical.We also can use scientific notation converter to find the number.